Ruota o mandala delle tabelline Montessori – Il bambino può avere già osservato, in natura, ordini numerici in determinati sistemi, per esempio nelle piante la specifica disposizione dei petali e dei sepali o delle antere: è facile per lui scoprire la regolarità di un disegno anche nelle tabelline.
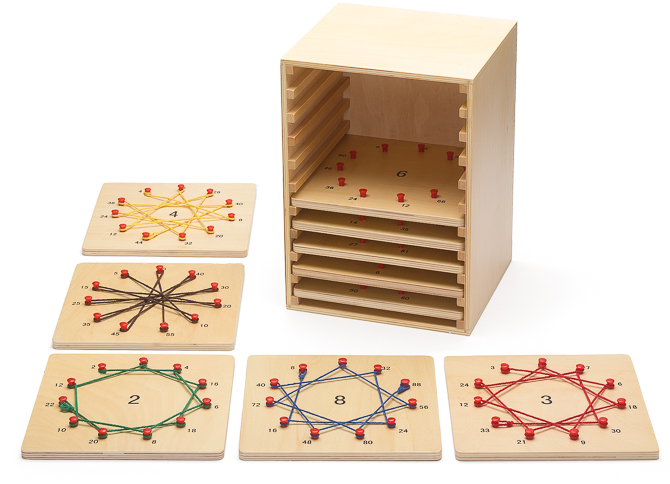

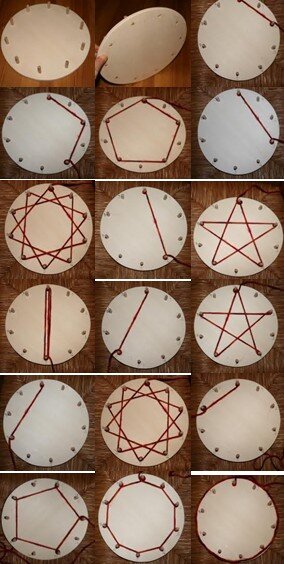
Costruire la ruota delle tabelline è molto semplice ed economico. Noi abbiamo ritagliato dei dischi di compensato del diametro di circa 25cm. Poi abbiamo praticato col trapano dieci fori lungo il bordo, a distanza regolare, e vi abbiamo inserito ed incollato dei tasselli di legno. I bambini li hanno rifiniti con la carta vetrata.
Senza trapano e tasselli, si possono anche più semplicemente piantare sul retro dieci chiodi con la testa grossa, lasciandoli sporgere sul davanti (per la punta) per almeno un centimetro.
Al legno può naturalmente essere sostituito del cartone spesso, più semplice da ritagliare)



I tasselli (o i chiodi) vengono quindi numerati da 0 a 9. Come verrà spiegato meglio di seguito, nel cerchio vengono prese in considerazione solo le unità, mentre le decine bisogna ricordarle. Se ad esempio dobbiamo segnare il numero 16 useremo il chiodino 6, per il 63 il chiodino 3, ecc…
Ruota o mandala delle tabelline Montessori
Tabellina del 2
Il filo di lana sullo 0, sul 2, sul 4, sul 6, sull’8, sullo 0 (10), poi ancora sul 2 (12), sul 4 (14), sul 6 (16), sull’8 (18) e ancora sullo 0 (20)


Così, mentre il bambino ripete oralmente la tabellina, sulla ruota si crea questo:

Ruota o mandala delle tabelline Montessori
Tabellina del 3
Il filo di lana sullo 0, sul 3, sul 6, sul 9, sul 2 (12), sul 5 (15), sull’8 (18), sull’1 (21), sul 4 (24), sul 7 (27) e di nuovo sullo 0 (30)

Così, mentre il bambino ripete oralmente la tabellina, sulla ruota si crea questo:

Ruota o mandala delle tabelline Montessori
Tabellina del 4
Filo di lana sullo 0, sul 4, sull’8, sul 2 (12), sul 6 (16), ancora sullo 0 (20), poi ancora sul 4, sull’8, sul 2, sul 6 e infine di nuovo sullo 0 (40)

Questa (come naturalmente quella del 6) è la tabellina preferita dai bambini, perchè durante l’esecuzione il disegno risulta molto scomposto, ma al termine dell’esercizio, ripetendo oralmente la tabellina si ottiene questa forma:

Ruota o mandala delle tabelline Montessori
tabellina del 5
Filo di lana una volta sullo 0 e una volta sul 5, fino ad arrivare al 50.

La tabellina del 5 mostra come il 5 è la metà del cerchio, cioè del 10.
Ruota o mandala delle tabelline Montessori
Tabellina del 6
Anche con la tabellina del 6 si ottiene la stella a 5 punte, come con quella del 4 (infatti 6+4=10), con andamento inverso del filo di lana:


Ruota o mandala delle tabelline Montessori
Tabellina del 7
Allo stesso modo, ripetendo la tabellina del 7 sulla ruota, si ottiene la stella a 10 punte, come per la tabellina del 3 (infatti 7+3=10)


Ruota o mandala delle tabelline Montessori
Tabellina dell’8
E ripetendo quella dell’8, si torna al pentagono, come per la tabellina del 2 (8+2=10)


Ruota o mandala delle tabelline Montessori
Tabellina del 9
ripetendo la tabellina del 9, si vede bene come questa tabellina , da alcuni considerata “difficile”, si memorizza facilmente procedendo di numero in numero aggiungendo una decina e togliendo una unità:

Ruota o mandala delle tabelline Montessori
Tabellina del 10
La tabellina del 10 è il cerchio:

Con lo stesso principio si possono preparare dei cubi per sedersi in cerchio, numerati sempre da 0 a 9:

Ruota o mandala delle tabelline Montessori

Montessori wheel or mandala of multiplication tables – The child may have already noted, in nature, numerical orders in certain systems, for example in plants the specific arrangement of petals and sepals, or anthers: it’s easy for them to find out the regularity of a pattern even in the multiplication tables.

Building the wheel of multiplication tables is very simple and cheap. We have cut some disks of plywood of diameter about 25cm. Then we practiced with drill ten holes along the edge, at regular distances, and we have inserted and glued dowels of wood. The children have finished with the sandpaper.
Without drill and dowels, you can also simply plant on the back ten nails with a big head, letting them stick out from the front (for the tip) for at least one centimeter.
Wood can be replaced with thick cardboard, easier to trim.



The dowels (or nails) are then numbered from 0 to 9. As will be explained below, in the circle are taken into account only the units, while the tens must remember. For example, if we have to mark the number 16 we will use the nail 6, to 63 the nail 3, etc …
Multiplication table of 2 The wool thread on the 0, on the 2, on the 4, on the 6, on the 8, on the 0 (10), then again on the 2 (12), on the 4 (14), on the 6 (16), on the 8 (18) and on the 0 (20)


So, while the child repeats the multiplication table orally, on the wheel are creating this:

Multiplication table of 3
The wool thread on the 0, on the 3, on the 6, on the 9, on the 2 (12), on the 5 (15), on the 8 (18), on the 1 (21), on the 4 (24), on the 7 (27) and on the 0 (30)

So, while the child repeats the multiplication table orally, on the wheel are creating this:

Multiplication table of 4
The wool thread on the 0, on the 4, on the 8, on the 2 (12), on the 6 (16), on the 0 (20), on the 4, on the 8, on the 2, on the 6 and on the 0 (40)

This (of course as that of 6) is the multiplication table preferred by children, because when running the design is very decomposed, but at the end exercise, orally repeating the multiplication table is obtained this form:

Multiplication table of 5
Wool thread once on the 0 and once on the 5, up to the 50.

The multiplication table of 5, shows how the 5 is half of the circle, that is, 10.
Multiplication table of 6 Even with the multiplication table of 6 is obtained the 5-pointed star, as with that of 4 (that is 6 + 4 = 10), with the inverse of the wool yarn:


Multiplication table of 7 Similarly, by repeating the multiplication table of 7 on the wheel, you get the star at 10 bits, as for the multiplication table of 3 (in fact 7 + 3 = 10)


Multiplication table of 8
And repeating that of 8, we return to the pentagon, as the multiplication table of 2 (8 + 2 = 10):


Multiplication table of 9
repeating the multiplication table of 9, one can see how this multiplication table, by some considered “difficult”, you learn easily progressing in number in number, adding 1 ten, and removing one unit:

Multiplication table of 10
The multiplication table of 10 is the circle:

With the same principle can be prepared cubes to sit in a circle, always numbered from 0 to 9:




